Tessellations have been studied for millennia, and analysis of 2d shapes' tessellations is essentially a closed problem. We know which shapes, when repeated over a 2d plane, completely fill the space. You may be familiar with the beautiful tilings drawn by M. C. Escher (see here, for instance). However, in three dimensions, we have made far less progress, and although we can generalize the 2d case from regular polygons to arbitrary shapes, this is inaccessible to us.

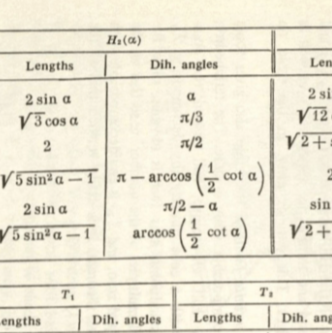
Given that they are the simplest 3d polyhedron, tetrahedra (4 faces, all triangles) were chosen to explore tessellation in 3d. The central problem is: Which tetrahedra can fill 3d-space entirely? From there, interesting problems (15, at the time I joined the project) were created, spanning multiple fields. Some were programming-based (generate code to check properties of tetrahedra); others involved finding relationships between dihedral angles (the angle between two planes whence tetrahedral faces come).

I worked with a group of 3-4 MIT undergraduates to unpack the mysteries of 3d research. Other than the mathematics exploration I had performed as a child between ages 6 to 11, this was my first mathematics research exposure. I relied on my knowledge of group theory and algebra from Peter J. Cameron's excellent volume, "Introduction to Algebra", to make progress on the problems. The research was supervised by Prof. Bjorn Poonen.

The project was split into three rough components. Most of the time was taken by reading and scouting relevant information from the massive material trove of 18.TETRA (the affectionate name given to our project). Textbooks, articles, and research spanning multiple decades were consulted; there were even a few articles in German (which I can understand). Once the right "pieces of the puzzle" were found, I worked alone to fit them together. There is no real way to describe this; one has to perform mathematical research to feel that way. Finally, we had several group meetings a week, to go over findings and patch up the missing links in each others' work.

The takeaways from my first true mathematics research experience revolved around one aspect: structure. Despite my knowledge of group theory, and my acquisition of knowledge through the large amount of textbooks and resources consulted, I had not marshalled them in the optimal way. This is because I did not fully grasp the structure of research. Whereas mathematics competitions and self-exploration were about simple strategies—taking the correct approach off the metaphorical toolbox and using it, and just reading, reading, reading, respectively—research was far more complicated. Rather than having already-solved problems handed to us in bite-size pieces to challenge us, or expanding out on our own without a clear problem, we had defined problems without known solutions, and it was our job to find an answer, similar to how a Smurf invents a gadget that would take him to the moon¹. In my view, there are few clear principles that can make research effective. One has to learn along the way, and experience is the only true guide. Discussing research philosophy is less fruitful than performing research oneself.

Footnotes

¹In fact, the Smurfs never went to the moon, though they came distantly "close" in "The Astrosmurf". It is Dreamy's birthday, and he wishes to go to another planet. The Smurfs fulfill this by using magical potions to alter Earth's terrain and turn themselves into Swoofs.